Design a Reliable and Accurate Op Amp Driver and SAR ADC Combination for Precision Medical Imaging
Contributed By DigiKey's North American Editors
2019-09-04
Medical imaging applications such as MRIs, ultrasound scanners, and x-ray equipment depend on increasing amounts of accurate data, particularly as devices and systems become more connected. However, the accuracy of data depends upon good front-end design to acquire the sensor signal while minimizing instability due to noise before the sensed signal is converted to the digital domain.
The problem of stability is partly addressed using a differential input, successive approximation register (SAR) analog-to-digital converter (ADC) to provide accurate digital results for a given analog input signal. However, if the input signal is unstable due to noise, the converter can only reliably produce the input signal’s noise. The challenge is to ensure the analog system noise and operational amplifier (op amp) bandwidth complement the SAR ADC.
This article briefly discusses the proper selection of the complementary op amp and high-resolution SAR ADC. It will then introduce a SAR ADC and a fully differential amplifier from Analog Devices and show how to combine them to reach 16-bit signal-to-noise ratio (SNR) and total harmonic distortion (THD) performance.
Medical imaging performance requirements
When working with imaging medical equipment, every output result has a seismic impact on the ability of the doctor to evaluate and prescribe effective treatments. Whether the medical equipment is an MRI, ultrasound scanner, or x-ray unit, a journey from symptoms to a reasonable action can originate with the equipment’s results and doctor’s evaluation. High performance medical equipment improves image quality and output results. An improvement in equipment sensitivity reduces patient exposure, unnecessary repetitive testing, and improves diagnostic image quality.
At the component level, the equipment’s amplifiers, ADCs, and their implementation define the ultimate level of sensitivity and image quality. These systems require 16-bit performance from the analog-to-digital conversion process to ensure that the image quality is retained at the output level. As a starting place for analog and digital systems, this 16-bit resolution translates to a typical system performance >98 decibels (dB) SNR and < -107.5 THD.
The SNR describes how much noise is riding on top of a signal. SNR excludes harmonic signals and DC. The ideal SNR for a SAR ADC converter with a full-scale sine wave input is (6.02 x n) +1.76 dB, where n is the number of converter bits. THD is the rms sum of the powers of the harmonic components (spurs) at a multiple of the input signal, ratioed to the input signal power. This ratio is specified in rms decibels (dB).
The required performance can be achieved using the Analog Devices ADA4945-1ACPZ-R2 op amp and the AD4003BCPZ-RL7 SAR ADC (Figure 1). The ADA4945-1ACPZ-R2 is a low-noise, fully differential, high-speed op amp in a unity gain configuration. This effectively drives high-resolution SAR ADCs. It operates over a broad power supply range (3 to 10 volts) and has low offset voltage as well as low noise of 1.8 nanovolts per root Hertz (nV√Hz) @ 100 kilohertz (kHz). The AD4003BCPZ-RL7 is an 18-bit, 2 megasample/second (MSPS) differential input SAR ADC, with a typical SNR equaling 100.5 dB, a THD of -123 dB, and integral non-linearity (INL) of ±1.0 least significant bit (LSB).
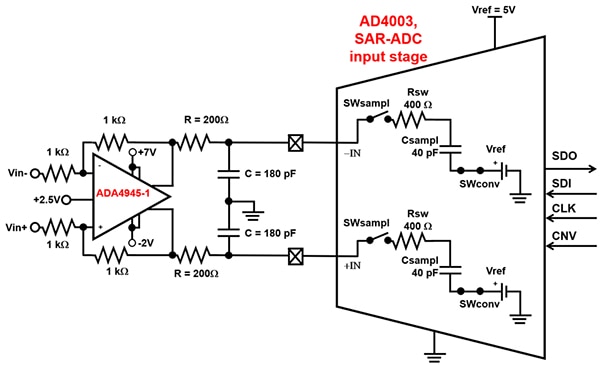 Figure 1: Simplified medical imaging data acquisition circuit based on Analog Devices’ ADA4945-1ACPZ-R2 op amp and an AD4003BCPZ-RL7 SAR ADC. (Image source: Bonnie Baker)
Figure 1: Simplified medical imaging data acquisition circuit based on Analog Devices’ ADA4945-1ACPZ-R2 op amp and an AD4003BCPZ-RL7 SAR ADC. (Image source: Bonnie Baker)
System noise analysis
A key design goal for precision medical systems is to achieve a high SNR. The way to improve SNR is to both select low-noise components and to increase the full-scale signal amplitude (Figure 2).
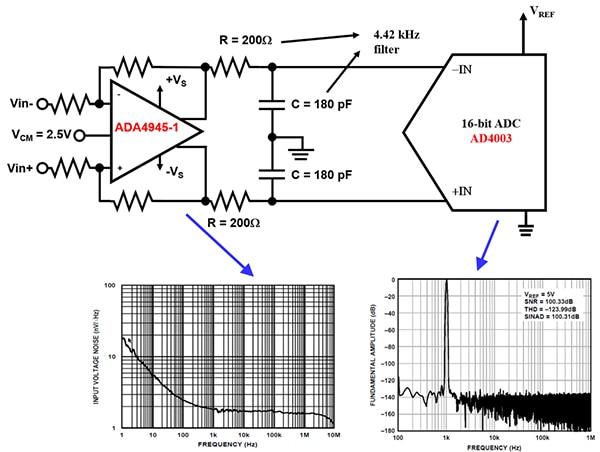 Figure 2: The units for noise specifications in the analog domain are in terms of time and frequency. The units for noise specifications in the digital domain are in terms of dB. (Image source: Bonnie Baker, based on material from Analog Devices)
Figure 2: The units for noise specifications in the analog domain are in terms of time and frequency. The units for noise specifications in the digital domain are in terms of dB. (Image source: Bonnie Baker, based on material from Analog Devices)
In Figure 1, the ADA4945-1 amplifier’s power supply is wide enough to ensure undistorted rail-to-rail output performance. The AD4003 SAR ADC 5 volt reference covers the input range. The key to choosing the correct components is to understand the total noise power of the signal chain’s components.
Note that the bottom plots in Figure 2 have differing units. In the analog domain, the units of measure for noise is V/√Hz. Noise in the digital domain is measured in dB. As shown, the noise specification units between the analog and digital domain differ.
Op amp noise
In the analog domain, the units of measure for noise is also given as volts-rms for a statistical mean across a given bandwidth. For instance, the differential input voltage noise of the ADA4945-1 is 5 nV/√Hz @ 5 Hz and 1.8 nV/√Hz @ 100 kHz (Figure 3).
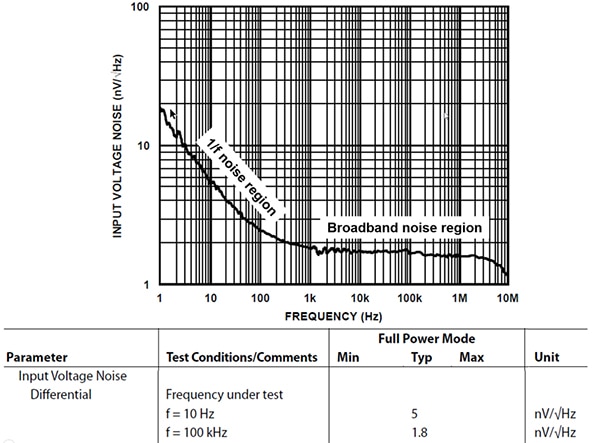 Figure 3: The frequency vs. input voltage noise plot of the ADA4945-1 amplifier showing the amplifier’s 1/f and broadband noise regions. (Image source: Bonnie Baker, based on material from Analog Devices)
Figure 3: The frequency vs. input voltage noise plot of the ADA4945-1 amplifier showing the amplifier’s 1/f and broadband noise regions. (Image source: Bonnie Baker, based on material from Analog Devices)
In Figure 3, the challenge with respect to the two noise regions is to combine them into one noise statistical average. The referred-to-input 1/f region rms noise can be found using Equation 1:
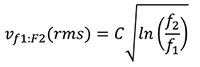 Equation 1
Equation 1
Where C is the amplifier’s noise density at 1 Hz, and f1 and f2 define the bandwidth of the 1/f region. Typically, f1 is equal to 0.1 Hz.
Putting in the numbers:
f1 = 0.1 Hz
f2 = 1 kHz
C = 19 nV/√Hz
The rms noise of the ADA4945-1 in the 1/f region is 57.66 nV rms
The ADA4945-1’s broadband rms noise referred to input is calculated using Equation 2:
![]() Equation 2
Equation 2
Where en is the specified noise at a given frequency in the broadband region of the amplifier and BW is the bandwidth of the broadband region.
With:
en = 1.8 nV/√Hz
BW = 1 kHz to 4.42 megahertz (MHz) (Note: with 200 ohm (W), 180 picofarad (pF) low pass filter between the op amp and ADC)
The rms noise in the broadband region is 4.74 microvolts (mV) rms.
The total noise power present in any system is equal to the root-sum-square (RSS) of the noise power contributed by its individual component portions. The total amplifier referred-to-input noise is calculated using Equation 3:
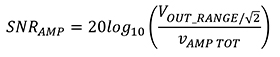 Equation 3
Equation 3
Where GAMP is equal to the amplifier gain.
With GAMP = 1, the total referred-to-output rms noise from the ADA4451 is 4.74 mV rms.
The analog domain calculation units for Equations 1, 2, and 3 are volts and frequency. The analog voltage conversion to a dB representation as SNR is equal to SNRAMP, as shown in Equation 4.
![]() Equation 4
Equation 4
Where VOUT_RANGE matches the SAR ADC input range.
With:
VOUT_RANGE = 9.5 volts
The SNRAMP from the ADA4451-2, referred to its output, is +123 dB.
Amplifier distortion
The ADA4945-1 is fabricated using Analog Devices’ proprietary, silicon germanium (SiGe) complementary bipolar process, enabling the device to achieve low levels of distortion.
With an input voltage range of -VS to (+VS – 1.3 volts), the second harmonic distortion (HD2) is equal to −133 decibels relative to the carrier frequency (dBc). HD2 and the third harmonic distortion (HD3) is −140 dBc HD3 at 1 kHz. At 100 kHz, HD2 equals −133 dBc and HD3 is −116 dBc.
SAR ADC noise
The derivation of the input referred noise for an amplifier comes from two frequency measurement points (1 Hz and 100 kHz). The derivation of an SAR-ADC signal to noise ratio is obtained using an FFT RSS calculation and is given in dB.
The ideal SNR of a SAR ADC is equal to (N x 6.02 + 1.76) dB, where N is equal to the number of converter bits. The ADA4003 SAR ADC is specified as an 18-bit converter, so the ideal SNR of this converter is equal to 110 dB. However, as shown later, the actual SNR of this device is equal to 100.3 dB.
The frequency spectrum of the SAR ADC’s FFT measurement spans from 0 to fs/2, where fs is equal to the converters sampling frequency (Figure 4).
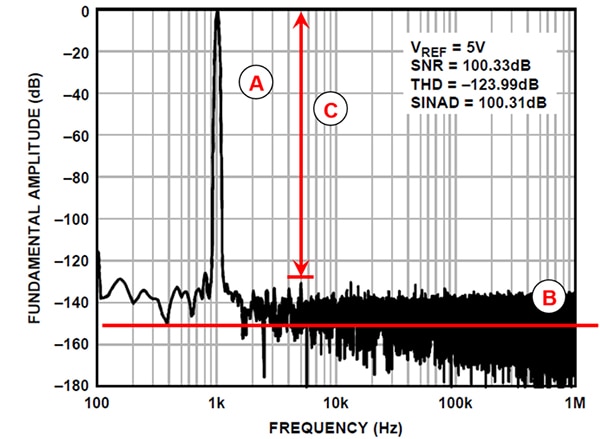 Figure 4: ADA4003 FFT data plot is used to calculate an ADC’s SNR and THD. (Image source: Bonnie Baker)
Figure 4: ADA4003 FFT data plot is used to calculate an ADC’s SNR and THD. (Image source: Bonnie Baker)
In Figure 4, the dominant spur (A) is the converter’s input signal. The (B) line shows the output noise from the converter which includes quantization and internal component noise. The secondary spur (C), which appears to be HD5, represents the dominant distortion at approximately -128 dB. All other spurs whose frequencies are multiples of the input signal (A) are added together with an RSS formula to generate the total THD value.
Combining SNR and THD: SINAD
A figure of merit (FoM) to explore is SNR plus distortion (SINAD, or SNR+D). This term can also be THD + noise. SINAD is the calculated combination of SNR and THD, or the ratio of the rms amplitude of the fundamental input signal to the rms sum of all other spectral components below one-half of the sampling frequency (excluding DC). The theoretical minimum for SINAD is equal to the ideal SNR, or 6.02n + 1.76 dB with SAR and pipeline converters.
SINAD is either given in dBc when the absolute power of the fundamental is used as the reference, or decibels relative to full-scale (dBFS) when the power of the fundamental is extrapolated to the converter full-scale range.
SINAD is a critical specification in designs for digital oscilloscope/waveform recorders, as well as geophysical image processing, radar, sonar, spectrum analysis, video telecommunication, and wideband digital receiver applications.
Combined noise and distortion
Going back to the original design, the system requirement is for a 16-bit system. This 16-bit resolution translates to a typical system performance of >98 dB SNR and < -107.5 THD.
Now it is time to combine all SNR and THD amplifier and SAR ADC errors into one FoM. The amplifier and SAR ADC noise are combined to determine the total system noise using Equation 5:
![]() Equation 5
Equation 5
In Equation 5, the two SNR terms with units of dB cannot be added together. The amplifier and SAR ADC SNR terms are converted to a linear ratio. Once this is complete, these terms are added together and then changed back to decibels.
The amplifier and SAR ADC distortion are combined to determine the total system distortion using Equation 6:
![]() Equation 6
Equation 6
The system’s SNR is combined with the system’s THD using Equation 7:
![]() Equation 7
Equation 7
At 1 kHz and 10 kHz signal frequencies, the tested SNR and THD for the combination of the ADA1945-1 amplifier driving the AD4003 SAR ADC meet the required >98 dB SNR and < -107.5 THD (Table 1).
|
Table 1: A summary of the ADA4945-1and AD4003 per Figure 1. At 100 kHz, the ADA4945-1 is able to sustain 16-bit performance, where the AD4003 SNR and THD start to degrade. (Table source: Bonnie Baker)
At 100 kHz, the ADA4945-1 is able to sustain 16-bit performance, where the AD4003 SNR and THD start to degrade.
Conclusion
The combination of a fully differential amplifier and 18-bit SAR ADC are required to create a high-precision, 16-bit system for MRIs, ultrasound scanners, and x-ray systems. To deliver the best overall performance, the Analog Devices ADA4945-1 and AD4003 are a good match for a low-noise, low-distortion solution for medical instrumentation systems.

Disclaimer: The opinions, beliefs, and viewpoints expressed by the various authors and/or forum participants on this website do not necessarily reflect the opinions, beliefs, and viewpoints of DigiKey or official policies of DigiKey.